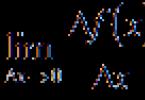When deriving the very first formula of the table, we will proceed from the definition of the derivative function at a point. Let's take where x– any real number, that is, x– any number from the domain of definition of the function. Let us write down the limit of the ratio of the increment of the function to the increment of the argument at : 
It should be noted that under the limit sign the expression is obtained, which is not the uncertainty of zero divided by zero, since the numerator does not contain an infinitesimal value, but precisely zero. In other words, the increment of a constant function is always zero.
Thus, derivative of a constant functionis equal to zero throughout the entire domain of definition.
Derivative of a power function.
The formula for the derivative of a power function has the form 
, where the exponent p– any real number.
Let us first prove the formula for the natural exponent, that is, for p = 1, 2, 3, …
We will use the definition of derivative. Let us write down the limit of the ratio of the increment of a power function to the increment of the argument: 
To simplify the expression in the numerator, we turn to the Newton binomial formula: 
Hence, 
This proves the formula for the derivative of a power function for a natural exponent.
Derivative of an exponential function.
We present the derivation of the derivative formula based on the definition:
We have arrived at uncertainty. To expand it, we introduce a new variable, and at . Then . In the last transition, we used the formula for transitioning to a new logarithmic base.
Let's substitute into the original limit: 
If we recall the second remarkable limit, we arrive at the formula for the derivative of the exponential function: 
Derivative of a logarithmic function.
Let us prove the formula for the derivative of a logarithmic function for all x from the domain of definition and all valid values of the base a logarithm By definition of derivative we have: 
As you noticed, during the proof the transformations were carried out using the properties of the logarithm. Equality 
is true due to the second remarkable limit.
Derivatives of trigonometric functions.
To derive formulas for derivatives of trigonometric functions, we will have to recall some trigonometry formulas, as well as the first remarkable limit.
By definition of the derivative for the sine function we have 
.
Let's use the difference of sines formula: 
It remains to turn to the first remarkable limit: 
Thus, the derivative of the function sin x There is cos x.
The formula for the derivative of the cosine is proved in exactly the same way. 
Therefore, the derivative of the function cos x There is –sin x.
We will derive formulas for the table of derivatives for tangent and cotangent using proven rules of differentiation (derivative of a fraction). 
Derivatives of hyperbolic functions.
The rules of differentiation and the formula for the derivative of the exponential function from the table of derivatives allow us to derive formulas for the derivatives of the hyperbolic sine, cosine, tangent and cotangent. 
Derivative of the inverse function.
To avoid confusion during presentation, let's denote in subscript the argument of the function by which differentiation is performed, that is, it is the derivative of the function f(x) By x.
Now let's formulate rule for finding the derivative of an inverse function.
Let the functions y = f(x) And x = g(y) mutually inverse, defined on the intervals and respectively. If at a point there is a finite non-zero derivative of the function f(x), then at the point there is a finite derivative of the inverse function g(y), and 
. In another post 
.
This rule can be reformulated for any x from the interval , then we get 
.
Let's check the validity of these formulas.
Let's find the inverse function for the natural logarithm 
(Here y is a function, and x- argument). Having resolved this equation for x, we get (here x is a function, and y– her argument). That is, 
and mutually inverse functions.
From the table of derivatives we see that 
And 
.
Let’s make sure that the formulas for finding the derivatives of the inverse function lead us to the same results: 
As you can see, we got the same results as in the derivatives table.
Now we have the knowledge to prove formulas for the derivatives of inverse trigonometric functions.
Let's start with the derivative of the arcsine.
. Then, using the formula for the derivative of the inverse function, we get
All that remains is to carry out the transformations.
Since the arcsine range is the interval 
, That 
(see the section on basic elementary functions, their properties and graphs). Therefore, we are not considering it.
Hence, 
. The domain of definition of the arcsine derivative is the interval (-1;
1)
.
For the arc cosine, everything is done in exactly the same way: 
Let's find the derivative of the arctangent.
For the inverse function is 
.
Let's express the arctangent in terms of arccosine to simplify the resulting expression.
Let arctgx = z, Then 
Hence, 
The derivative of the arc cotangent is found in a similar way: 
The process of finding the derivative of a function is called differentiation. The derivative has to be found in a number of problems in the course of mathematical analysis. For example, when finding extremum points and inflection points of a function graph.
How to find?
To find the derivative of a function you need to know the table of derivatives of elementary functions and apply the basic rules of differentiation:
- Moving the constant beyond the sign of the derivative: $$ (Cu)" = C(u)" $$
- Derivative of the sum/difference of functions: $$ (u \pm v)" = (u)" \pm (v)" $$
- Derivative of the product of two functions: $$ (u \cdot v)" = u"v + uv" $$
- Derivative of a fraction: $$ \bigg (\frac(u)(v) \bigg)" = \frac(u"v - uv"))(v^2) $$
- Derivative of a complex function: $$ (f(g(x)))" = f"(g(x)) \cdot g"(x) $$
Examples of solutions
| Example 1 |
| Find the derivative of the function $ y = x^3 - 2x^2 + 7x - 1 $ |
| Solution |
|
The derivative of the sum/difference of functions is equal to the sum/difference of derivatives: $$ y" = (x^3 - 2x^2 + 7x - 1)" = (x^3)" - (2x^2)" + (7x)" - (1)" = $$ Using the rule for the derivative of a power function $ (x^p)" = px^(p-1) $ we have: $$ y" = 3x^(3-1) - 2 \cdot 2 x^(2-1) + 7 - 0 = 3x^2 - 4x + 7 $$ It was also taken into account that the derivative of a constant is equal to zero. If you cannot solve your problem, then send it to us. We will provide detailed solution. You will be able to view the progress of the calculation and gain information. This will help you get your grade from your teacher in a timely manner! |
| Answer |
| $$y" = 3x^2 - 4x + 7 $$ |
WITH editing materials on the topic “derivative”. Basic school level.
Theoretical information for students, teachers and tutors in mathematics. To help conduct classes.
Definition: the derivative of a function at a point is the limit of the ratio of the increment of the function to the increment of the variable, that is
Table of derivatives of basic mathematical functions:
Rules for calculating derivatives
Derivative of a sum any two expressions is equal to the sum of the derivatives of these expressions (the derivative of the sum is equal to the sum of the derivatives)
Derivative of the difference any two expressions is equal to the difference of the derivatives of these terms (the derivative of the difference is equal to the difference of the derivatives).
Derivative of the product two factors is equal to the product of the derivative of the first factor and the second plus the product of the first factor and the derivative of the second (the sum of the derivatives of the factors taken in turn).
Math tutor comment: When I briefly remind a student about the rule for calculating the derivative of a product, I say this: derivative of the first factor by the second plus exchange strokes!
Derivative of quotient two expressions is equal to the quotient of the difference between the derivatives of the factors taken in turn and the square of the denominator.
Derivative of the product of a number and a function. To find the derivative of the product of a number and a literal expression (function), you need to multiply this number by the derivative of this literal expression.
Derivative of a complex function:
To calculate the derivative of a complex function, you need to find the derivative of the outer function and multiply it by the derivative of the inner function.
Your comments and feedback on the derivatives page:
Alexander S.
I really needed a table. One of the most on the Internet. Thank you very much for the explanations and rules too. At least one more example would be great for them. Thank you very much again.
Kolpakov A.N., mathematics tutor: ok, I’ll try to update the page with examples in the near future.
Virtual mathematical reference book.
Kolpakov Alexander Nikolaevich, mathematics tutor.
- Table of derivatives of exponential and logarithmic functions
Derivatives of simple functions
1. The derivative of a number is zeroс´ = 0
Example:
5´ = 0
Explanation:
The derivative shows the rate at which the value of a function changes when its argument changes. Since the number does not change in any way under any conditions, the rate of its change is always zero.
2. Derivative of a variable equal to one
x´ = 1
Explanation:
With each increment of the argument (x) by one, the value of the function (the result of the calculation) increases by the same amount. Thus, the rate of change in the value of the function y = x is exactly equal to the rate of change in the value of the argument.
3. The derivative of a variable and a factor is equal to this factor
сx´ = с
Example:
(3x)´ = 3
(2x)´ = 2
Explanation:
In this case, every time the function argument changes ( X) its value (y) increases in With once. Thus, the rate of change of the function value in relation to the rate of change of the argument is exactly equal to the value With.
Whence it follows that
(cx + b)" = c
that is, the differential of the linear function y=kx+b is equal to the slope of the line (k).
4. Modulo derivative of a variable equal to the quotient of this variable to its modulus
|x|"= x / |x| provided that x ≠ 0
Explanation:
Since the derivative of a variable (see formula 2) is equal to one, the derivative of the module differs only in that the value of the rate of change of the function changes to the opposite when crossing the point of origin (try drawing a graph of the function y = |x| and see for yourself. This is exactly what value and returns the expression x / |x|. When x< 0 оно равно (-1), а когда x >0 - one. That is, for negative values of the variable x, with each increase in the argument, the value of the function decreases by exactly the same value, and for positive values, on the contrary, it increases, but by exactly the same value.
5. Derivative of a variable to a power equal to the product of a number of this power and a variable to the power reduced by one
(x c)"= cx c-1, provided that x c and cx c-1 are defined and c ≠ 0
Example:
(x 2)" = 2x
(x 3)" = 3x 2
To remember the formula:
Move the degree of the variable down as a factor, and then reduce the degree itself by one. For example, for x 2 - the two was ahead of the x, and then the reduced power (2-1 = 1) simply gave us 2x. The same thing happened for x 3 - we “move down” the triple, reduce it by one and instead of a cube we have a square, that is, 3x 2. A little "unscientific" but very easy to remember.
6.Derivative of a fraction 1/x
(1/x)" = - 1 / x 2
Example:
Since a fraction can be represented as raising to a negative power
(1/x)" = (x -1)", then you can apply the formula from rule 5 of the table of derivatives
(x -1)" = -1x -2 = - 1 / x 2
7. Derivative of a fraction with a variable of arbitrary degree in the denominator
(1 / x c)" = - c / x c+1
Example:
(1 / x 2)" = - 2 / x 3
8. Derivative of the root(derivative of variable under square root)
(√x)" = 1 / (2√x) or 1/2 x -1/2
Example:
(√x)" = (x 1/2)" means you can apply the formula from rule 5
(x 1/2)" = 1/2 x -1/2 = 1 / (2√x)
9. Derivative of a variable under the root of an arbitrary degree
(n √x)" = 1 / (n n √x n-1)
Date: 05/10/2015
How to find the derivative?
Rules of differentiation.
To find the derivative of any function, you need to master only three concepts:
2. Rules of differentiation.
3. Derivative of a complex function.
Exactly in that order. It's a hint.)
Of course, it would be nice to have an idea about derivatives in general). What a derivative is and how to work with the table of derivatives is clearly explained in the previous lesson. Here we will deal with the rules of differentiation.
Differentiation is the operation of finding the derivative. There is nothing more hidden behind this term. Those. expressions "find the derivative of a function" And "differentiate a function"- It is the same.
Expression "rules of differentiation" refers to finding the derivative from arithmetic operations. This understanding helps a lot to avoid confusion in your head.
Let's concentrate and remember all, all, all arithmetic operations. There are four of them). Addition (sum), subtraction (difference), multiplication (product), and division (quotient). Here they are, the rules of differentiation:
The plate shows five rules on four arithmetic operations. I didn’t get shortchanged.) It’s just that rule 4 is an elementary consequence of rule 3. But it is so popular that it makes sense to write (and remember!) it as an independent formula.
Under the designations U And V some (absolutely any!) functions are implied U(x) And V(x).
Let's look at a few examples. First - the simplest ones.
Find the derivative of the function y=sinx - x 2
Here we have difference two elementary functions. We apply rule 2. We will assume that sinx is a function U, and x 2 is the function V. We have every right to write:
y" = (sinx - x 2)" = (sinx)"- (x 2)"
That’s better, right?) All that remains is to find the derivatives of sine and square of x. There is a table of derivatives for this purpose. We just look for the functions we need in the table ( sinx And x 2), look at what derivatives they have and write down the answer:
y" = (sinx)" - (x 2)" = cosx - 2x
That's it. Rule 1 of sum differentiation works exactly the same.
What if we have several terms? No problem.) We break the function into terms and look for the derivative of each term independently of the others. For example:
Find the derivative of the function y=sinx - x 2 +cosx - x +3
We boldly write:
y" = (sinx)" - (x 2)" + (cosx)" - (x)" + (3)"
At the end of the lesson I will give tips to make life easier when differentiating.)
Practical tips:
1. Before differentiation, see if it is possible to simplify the original function.
2. In complicated examples, we describe the solution in detail, with all the parentheses and dashes.
3. When differentiating fractions with a constant number in the denominator, we turn division into multiplication and use rule 4.
